Alan JoContinuous time Fourier transform
As → , for any finite time interval
As a polar form
If is real, magnitude is even function of and phase is an odd funtion of
Fourier transform
Inverse Fourier transform
Dot Product → orthogonal → below functions → these properties
Mixing linearity and time shifting make it easy to compute complex function’s FT like this
CTFT Properties
Derivation 하지말고 외워야
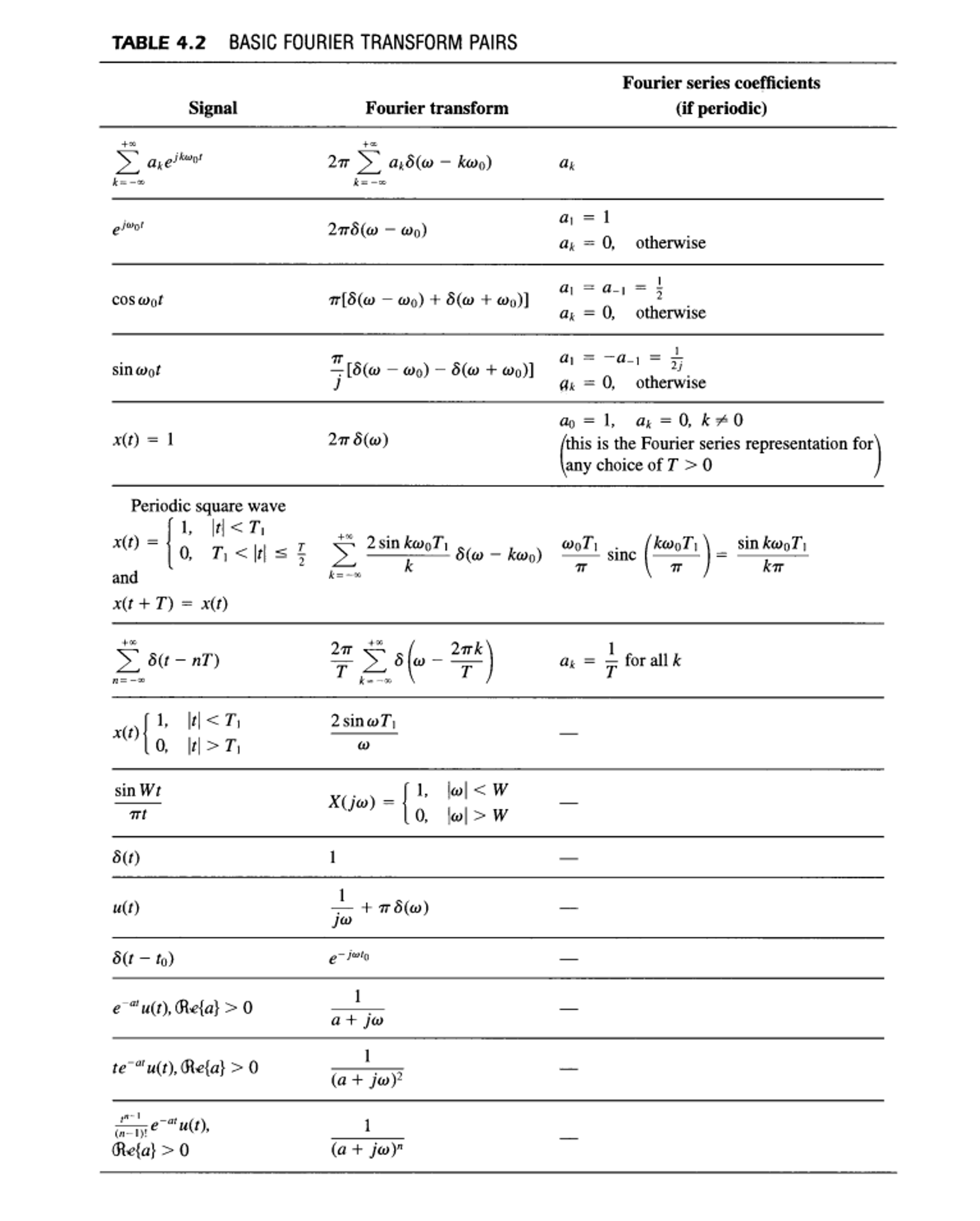
Fourier Transform Examples
